Tuesday 30 July 2019
Cave painting
Monday 29 July 2019
Aryabhata
Aryabhata (Sanskrit: आर्यभट, IAST: Āryabhaṭa) or Aryabhata I[2][3] (476–550 CE)[4][5] was the first of the major mathematician-astronomers from the classical age of Indian mathematics and Indian astronomy. His works include the Āryabhaṭīya (which mentions that in 3600 Kaliyuga, 499 CE, he was 23 years old)[6] and the Arya-siddhanta.
Place value system and zero
The place-value system, first seen in the 3rd-century Bakhshali Manuscript, was clearly in place in his work. While he did not use a symbol for zero, the French mathematician Georges Ifrah argues that knowledge of zero was implicit in Aryabhata's place-value system as a place holder for the powers of ten with null coefficients.[16]
However, Aryabhata did not use the Brahmi numerals. Continuing the Sanskritic tradition from Vedic times, he used letters of the alphabet to denote numbers, expressing quantities, such as the table of sines in a mnemonic form.[17]
Approximation of π
Aryabhata worked on the approximation for pi (π), and may have come to the conclusion that π is irrational. In the second part of the Aryabhatiyam (gaṇitapāda 10), he writes:
caturadhikaṃ śatamaṣṭaguṇaṃ dvāṣaṣṭistathā sahasrāṇām
ayutadvayaviṣkambhasyāsanno vṛttapariṇāhaḥ.
"Add four to 100, multiply by eight, and then add 62,000. By this rule the circumference of a circle with a diameter of 20,000 can be approached."
[18]
This implies that the ratio of the circumference to the diameter is ((4 + 100) × 8 + 62000)/20000 = 62832/20000 = 3.1416, which is accurate to five significant figures.[19]
It is speculated that Aryabhata used the word āsanna (approaching), to mean that not only is this an approximation but that the value is incommensurable (or irrational). If this is correct, it is quite a sophisticated insight, because the irrationality of pi (π) was proved in Europe only in 1761 by Lambert.[20]
After Aryabhatiya was translated into Arabic (c. 820 CE) this approximation was mentioned in Al-Khwarizmi's book on algebra.[9]
Trigonometry
In Ganitapada 6, Aryabhata gives the area of a triangle as
tribhujasya phalaśarīraṃ samadalakoṭī bhujārdhasaṃvargaḥ
that translates to: "for a triangle, the result of a perpendicular with the half-side is the area."[21]
Aryabhata discussed the concept of sine in his work by the name of ardha-jya, which literally means "half-chord". For simplicity, people started calling it jya. When Arabic writers translated his works from Sanskrit into Arabic, they referred it as jiba. However, in Arabic writings, vowels are omitted, and it was abbreviated as jb. Later writers substituted it with jaib, meaning "pocket" or "fold (in a garment)". (In Arabic, jiba is a meaningless word.) Later in the 12th century, when Gherardo of Cremona translated these writings from Arabic into Latin, he replaced the Arabic jaib with its Latin counterpart, sinus, which means "cove" or "bay"; thence comes the English word sine.[22]
Indeterminate equations
A problem of great interest to Indian mathematicians since ancient times has been to find integer solutions to Diophantine equations that have the form ax + by = c. (This problem was also studied in ancient Chinese mathematics, and its solution is usually referred to as the Chinese remainder theorem.) This is an example from Bhāskara's commentary on Aryabhatiya:
Find the number which gives 5 as the remainder when divided by 8, 4 as the remainder when divided by 9, and 1 as the remainder when divided by 7
That is, find N = 8x+5 = 9y+4 = 7z+1. It turns out that the smallest value for N is 85. In general, diophantine equations, such as this, can be notoriously difficult. They were discussed extensively in ancient Vedic text Sulba Sutras, whose more ancient parts might date to 800 BCE. Aryabhata's method of solving such problems, elaborated by Bhaskara in 621 CE, is called the kuṭṭaka (कुट्टक) method. Kuṭṭaka means "pulverizing" or "breaking into small pieces", and the method involves a recursive algorithm for writing the original factors in smaller numbers. This algorithm became the standard method for solving first-order diophantine equations in Indian mathematics, and initially the whole subject of algebra was called kuṭṭaka-gaṇita or simply kuṭṭaka.[23]
Algebra
In Aryabhatiya, Aryabhata provided elegant results for the summation of series of squares and cubes:[24]
{\displaystyle 1^{2}+2^{2}+\cdots +n^{2}={n(n+1)(2n+1) \over 6}} 1^2 + 2^2 + \cdots + n^2 = {n(n + 1)(2n + 1) \over 6}
and
{\displaystyle 1^{3}+2^{3}+\cdots +n^{3}=(1+2+\cdots +n)^{2}} 1^3 + 2^3 + \cdots + n^3 = (1 + 2 + \cdots + n)^2 (see squared triangular number)
AstronomyAryabhata is the author of several treatises on mathematics and astronomy, some of which are lost.
His major work, Aryabhatiya, a compendium of mathematics and astronomy, was extensively referred to in the Indian mathematical literature and has survived to modern times. The mathematical part of the Aryabhatiya covers arithmetic, algebra, plane trigonometry, and spherical trigonometry. It also contains continued fractions, quadratic equations, sums-of-power series, and a table of sines.
The Arya-siddhanta, a lost work on astronomical computations, is known through the writings of Aryabhata's contemporary, Varahamihira, and later mathematicians and commentators, including Brahmagupta and Bhaskara I. This work appears to be based on the older Surya Siddhanta and uses the midnight-day reckoning, as opposed to sunrise in Aryabhatiya. It also contained a description of several astronomical instruments: the gnomon (shanku-yantra), a shadow instrument (chhAyA-yantra), possibly angle-measuring devices, semicircular and circular (dhanur-yantra / chakra-yantra), a cylindrical stick yasti-yantra, an umbrella-shaped device called the chhatra-yantra, and water clocks of at least two types, bow-shaped and cylindrical.[9]
A third text, which may have survived in the Arabic translation, is Al ntf or Al-nanf. It claims that it is a translation by Aryabhata, but the Sanskrit name of this work is not known. Probably dating from the 9th century, it is mentioned by the Persian scholar and chronicler of India, Abū Rayhān al-Bīrūnī.[9]
Aryabhatiya
Main article: Aryabhatiya
Direct details of Aryabhata's work are known only from the Aryabhatiya. The name "Aryabhatiya" is due to later commentators. Aryabhata himself may not have given it a name. His disciple Bhaskara I calls it Ashmakatantra (or the treatise from the Ashmaka). It is also occasionally referred to as Arya-shatas-aShTa (literally, Aryabhata's 108), because there are 108 verses in the text. It is written in the very terse style typical of sutra literature, in which each line is an aid to memory for a complex system. Thus, the explication of meaning is due to commentators. The text consists of the 108 verses and 13 introductory verses, and is divided into four pādas or chapters:
Gitikapada: (13 verses): large units of time—kalpa, manvantra, and yuga—which present a cosmology different from earlier texts such as Lagadha's Vedanga Jyotisha (c. 1st century BCE). There is also a table of sines (jya), given in a single verse. The duration of the planetary revolutions during a mahayuga is given as 4.32 million years.
Ganitapada (33 verses): covering mensuration (kṣetra vyāvahāra), arithmetic and geometric progressions, gnomon / shadows (shanku-chhAyA), simple, quadratic, simultaneous, and indeterminate equations (kuṭṭaka).
Kalakriyapada (25 verses): different units of time and a method for determining the positions of planets for a given day, calculations concerning the intercalary month (adhikamAsa), kShaya-tithis, and a seven-day week with names for the days of week.
Golapada (50 verses): Geometric/trigonometric aspects of the celestial sphere, features of the ecliptic, celestial equator, node, shape of the earth, cause of day and night, rising of zodiacal signs on horizon, etc. In addition, some versions cite a few colophons added at the end, extolling the virtues of the work, etc.
The Aryabhatiya presented a number of innovations in mathematics and astronomy in verse form, which were influential for many centuries. The extreme brevity of the text was elaborated in commentaries by his disciple Bhaskara I (Bhashya, c. 600 CE) and by Nilakantha Somayaji in his Aryabhatiya Bhasya, (1465 CE).
The Aryabhatiya is also remarkable for its description of relativity of motion. He expressed this relativity thus: "Just as a man in a boat moving forward sees the stationary objects (on the shore) as moving backward, just so are the stationary stars seen by the people on earth as moving exactly towards the west."[15]
MathematicsName
While there is a tendency to misspell his name as "Aryabhatta" by analogy with other names having the "bhatta" suffix, his name is properly spelled Aryabhata: every astronomical text spells his name thus,[8] including Brahmagupta's references to him "in more than a hundred places by name".[1] Furthermore, in most instances "Aryabhatta" would not fit the meter either.[8]
Time and place of birth
Aryabhata mentions in the Aryabhatiya that he was 23 years old 3,600 years into the Kali Yuga, but this is not to mean that the text was composed at that time. This mentioned year corresponds to 499 CE, and implies that he was born in 476.[5] Aryabhata called himself a native of Kusumapura or Pataliputra (present day Patna, Bihar).[1]
Other hypothesis
Bhāskara I describes Aryabhata as āśmakīya, "one belonging to the Aśmaka country." During the Buddha's time, a branch of the Aśmaka people settled in the region between the Narmada and Godavari rivers in central India.[8][9]
It has been claimed that the aśmaka (Sanskrit for "stone") where Aryabhata originated may be the present day Kodungallur which was the historical capital city of Thiruvanchikkulam of ancient Kerala.[10] This is based on the belief that Koṭuṅṅallūr was earlier known as Koṭum-Kal-l-ūr ("city of hard stones"); however, old records show that the city was actually Koṭum-kol-ūr ("city of strict governance"). Similarly, the fact that several commentaries on the Aryabhatiya have come from Kerala has been used to suggest that it was Aryabhata's main place of life and activity; however, many commentaries have come from outside Kerala, and the Aryasiddhanta was completely unknown in Kerala.[8] K. Chandra Hari has argued for the Kerala hypothesis on the basis of astronomical evidence.[11]
Aryabhata mentions "Lanka" on several occasions in the Aryabhatiya, but his "Lanka" is an abstraction, standing for a point on the equator at the same longitude as his Ujjayini.[12]
Education
It is fairly certain that, at some point, he went to Kusumapura for advanced studies and lived there for some time.[13] Both Hindu and Buddhist tradition, as well as Bhāskara I (CE 629), identify Kusumapura as Pāṭaliputra, modern Patna.[8] A verse mentions that Aryabhata was the head of an institution (kulapa) at Kusumapura, and, because the university of Nalanda was in Pataliputra at the time and had an astronomical observatory, it is speculated that Aryabhata might have been the head of the Nalanda university as well.[8] Aryabhata is also reputed to have set up an observatory at the Sun temple in Taregana, Bihar.[14]
WorksBiographyBorn
476 CE
Kusumapura (Pataliputra) (present-day Patna, India)[1]
Died
550 CE
Residence
India
Academic background
Influences
Surya Siddhanta
Academic work
Era
Gupta era
Main interests
Mathematics, astronomy
Notable works
Āryabhaṭīya, Arya-siddhanta
Notable ideas
Explanation of lunar eclipse and solar eclipse, rotation of Earth on its axis, reflection of light by moon, sinusoidal functions, solution of single variable quadratic equation, value of π correct to 4 decimal places, diameter of Earth, calculation of the length of sidereal year
Influenced
Lalla, Bhaskara I, Brahmagupta, Varahamihira
For his explicit mention of the relativity of motion, he also qualifies as a major early physicist.[7]
The place-value system, first seen in the 3rd-century Bakhshali Manuscript, was clearly in place in his work. While he did not use a symbol for zero, the French mathematician Georges Ifrah argues that knowledge of zero was implicit in Aryabhata's place-value system as a place holder for the powers of ten with null coefficients.[16]
However, Aryabhata did not use the Brahmi numerals. Continuing the Sanskritic tradition from Vedic times, he used letters of the alphabet to denote numbers, expressing quantities, such as the table of sines in a mnemonic form.[17]
Approximation of π
Aryabhata worked on the approximation for pi (π), and may have come to the conclusion that π is irrational. In the second part of the Aryabhatiyam (gaṇitapāda 10), he writes:
caturadhikaṃ śatamaṣṭaguṇaṃ dvāṣaṣṭistathā sahasrāṇām
ayutadvayaviṣkambhasyāsanno vṛttapariṇāhaḥ.
"Add four to 100, multiply by eight, and then add 62,000. By this rule the circumference of a circle with a diameter of 20,000 can be approached."
[18]
This implies that the ratio of the circumference to the diameter is ((4 + 100) × 8 + 62000)/20000 = 62832/20000 = 3.1416, which is accurate to five significant figures.[19]
It is speculated that Aryabhata used the word āsanna (approaching), to mean that not only is this an approximation but that the value is incommensurable (or irrational). If this is correct, it is quite a sophisticated insight, because the irrationality of pi (π) was proved in Europe only in 1761 by Lambert.[20]
After Aryabhatiya was translated into Arabic (c. 820 CE) this approximation was mentioned in Al-Khwarizmi's book on algebra.[9]
Trigonometry
In Ganitapada 6, Aryabhata gives the area of a triangle as
tribhujasya phalaśarīraṃ samadalakoṭī bhujārdhasaṃvargaḥ
that translates to: "for a triangle, the result of a perpendicular with the half-side is the area."[21]
Aryabhata discussed the concept of sine in his work by the name of ardha-jya, which literally means "half-chord". For simplicity, people started calling it jya. When Arabic writers translated his works from Sanskrit into Arabic, they referred it as jiba. However, in Arabic writings, vowels are omitted, and it was abbreviated as jb. Later writers substituted it with jaib, meaning "pocket" or "fold (in a garment)". (In Arabic, jiba is a meaningless word.) Later in the 12th century, when Gherardo of Cremona translated these writings from Arabic into Latin, he replaced the Arabic jaib with its Latin counterpart, sinus, which means "cove" or "bay"; thence comes the English word sine.[22]
Indeterminate equations
A problem of great interest to Indian mathematicians since ancient times has been to find integer solutions to Diophantine equations that have the form ax + by = c. (This problem was also studied in ancient Chinese mathematics, and its solution is usually referred to as the Chinese remainder theorem.) This is an example from Bhāskara's commentary on Aryabhatiya:
Find the number which gives 5 as the remainder when divided by 8, 4 as the remainder when divided by 9, and 1 as the remainder when divided by 7
That is, find N = 8x+5 = 9y+4 = 7z+1. It turns out that the smallest value for N is 85. In general, diophantine equations, such as this, can be notoriously difficult. They were discussed extensively in ancient Vedic text Sulba Sutras, whose more ancient parts might date to 800 BCE. Aryabhata's method of solving such problems, elaborated by Bhaskara in 621 CE, is called the kuṭṭaka (कुट्टक) method. Kuṭṭaka means "pulverizing" or "breaking into small pieces", and the method involves a recursive algorithm for writing the original factors in smaller numbers. This algorithm became the standard method for solving first-order diophantine equations in Indian mathematics, and initially the whole subject of algebra was called kuṭṭaka-gaṇita or simply kuṭṭaka.[23]
Algebra
In Aryabhatiya, Aryabhata provided elegant results for the summation of series of squares and cubes:[24]
{\displaystyle 1^{2}+2^{2}+\cdots +n^{2}={n(n+1)(2n+1) \over 6}} 1^2 + 2^2 + \cdots + n^2 = {n(n + 1)(2n + 1) \over 6}
and
{\displaystyle 1^{3}+2^{3}+\cdots +n^{3}=(1+2+\cdots +n)^{2}} 1^3 + 2^3 + \cdots + n^3 = (1 + 2 + \cdots + n)^2 (see squared triangular number)
AstronomyAryabhata is the author of several treatises on mathematics and astronomy, some of which are lost.
His major work, Aryabhatiya, a compendium of mathematics and astronomy, was extensively referred to in the Indian mathematical literature and has survived to modern times. The mathematical part of the Aryabhatiya covers arithmetic, algebra, plane trigonometry, and spherical trigonometry. It also contains continued fractions, quadratic equations, sums-of-power series, and a table of sines.
The Arya-siddhanta, a lost work on astronomical computations, is known through the writings of Aryabhata's contemporary, Varahamihira, and later mathematicians and commentators, including Brahmagupta and Bhaskara I. This work appears to be based on the older Surya Siddhanta and uses the midnight-day reckoning, as opposed to sunrise in Aryabhatiya. It also contained a description of several astronomical instruments: the gnomon (shanku-yantra), a shadow instrument (chhAyA-yantra), possibly angle-measuring devices, semicircular and circular (dhanur-yantra / chakra-yantra), a cylindrical stick yasti-yantra, an umbrella-shaped device called the chhatra-yantra, and water clocks of at least two types, bow-shaped and cylindrical.[9]
A third text, which may have survived in the Arabic translation, is Al ntf or Al-nanf. It claims that it is a translation by Aryabhata, but the Sanskrit name of this work is not known. Probably dating from the 9th century, it is mentioned by the Persian scholar and chronicler of India, Abū Rayhān al-Bīrūnī.[9]
Aryabhatiya
Main article: Aryabhatiya
Direct details of Aryabhata's work are known only from the Aryabhatiya. The name "Aryabhatiya" is due to later commentators. Aryabhata himself may not have given it a name. His disciple Bhaskara I calls it Ashmakatantra (or the treatise from the Ashmaka). It is also occasionally referred to as Arya-shatas-aShTa (literally, Aryabhata's 108), because there are 108 verses in the text. It is written in the very terse style typical of sutra literature, in which each line is an aid to memory for a complex system. Thus, the explication of meaning is due to commentators. The text consists of the 108 verses and 13 introductory verses, and is divided into four pādas or chapters:
Gitikapada: (13 verses): large units of time—kalpa, manvantra, and yuga—which present a cosmology different from earlier texts such as Lagadha's Vedanga Jyotisha (c. 1st century BCE). There is also a table of sines (jya), given in a single verse. The duration of the planetary revolutions during a mahayuga is given as 4.32 million years.
Ganitapada (33 verses): covering mensuration (kṣetra vyāvahāra), arithmetic and geometric progressions, gnomon / shadows (shanku-chhAyA), simple, quadratic, simultaneous, and indeterminate equations (kuṭṭaka).
Kalakriyapada (25 verses): different units of time and a method for determining the positions of planets for a given day, calculations concerning the intercalary month (adhikamAsa), kShaya-tithis, and a seven-day week with names for the days of week.
Golapada (50 verses): Geometric/trigonometric aspects of the celestial sphere, features of the ecliptic, celestial equator, node, shape of the earth, cause of day and night, rising of zodiacal signs on horizon, etc. In addition, some versions cite a few colophons added at the end, extolling the virtues of the work, etc.
The Aryabhatiya presented a number of innovations in mathematics and astronomy in verse form, which were influential for many centuries. The extreme brevity of the text was elaborated in commentaries by his disciple Bhaskara I (Bhashya, c. 600 CE) and by Nilakantha Somayaji in his Aryabhatiya Bhasya, (1465 CE).
The Aryabhatiya is also remarkable for its description of relativity of motion. He expressed this relativity thus: "Just as a man in a boat moving forward sees the stationary objects (on the shore) as moving backward, just so are the stationary stars seen by the people on earth as moving exactly towards the west."[15]
MathematicsName
While there is a tendency to misspell his name as "Aryabhatta" by analogy with other names having the "bhatta" suffix, his name is properly spelled Aryabhata: every astronomical text spells his name thus,[8] including Brahmagupta's references to him "in more than a hundred places by name".[1] Furthermore, in most instances "Aryabhatta" would not fit the meter either.[8]
Time and place of birth
Aryabhata mentions in the Aryabhatiya that he was 23 years old 3,600 years into the Kali Yuga, but this is not to mean that the text was composed at that time. This mentioned year corresponds to 499 CE, and implies that he was born in 476.[5] Aryabhata called himself a native of Kusumapura or Pataliputra (present day Patna, Bihar).[1]
Other hypothesis
Bhāskara I describes Aryabhata as āśmakīya, "one belonging to the Aśmaka country." During the Buddha's time, a branch of the Aśmaka people settled in the region between the Narmada and Godavari rivers in central India.[8][9]
It has been claimed that the aśmaka (Sanskrit for "stone") where Aryabhata originated may be the present day Kodungallur which was the historical capital city of Thiruvanchikkulam of ancient Kerala.[10] This is based on the belief that Koṭuṅṅallūr was earlier known as Koṭum-Kal-l-ūr ("city of hard stones"); however, old records show that the city was actually Koṭum-kol-ūr ("city of strict governance"). Similarly, the fact that several commentaries on the Aryabhatiya have come from Kerala has been used to suggest that it was Aryabhata's main place of life and activity; however, many commentaries have come from outside Kerala, and the Aryasiddhanta was completely unknown in Kerala.[8] K. Chandra Hari has argued for the Kerala hypothesis on the basis of astronomical evidence.[11]
Aryabhata mentions "Lanka" on several occasions in the Aryabhatiya, but his "Lanka" is an abstraction, standing for a point on the equator at the same longitude as his Ujjayini.[12]
Education
It is fairly certain that, at some point, he went to Kusumapura for advanced studies and lived there for some time.[13] Both Hindu and Buddhist tradition, as well as Bhāskara I (CE 629), identify Kusumapura as Pāṭaliputra, modern Patna.[8] A verse mentions that Aryabhata was the head of an institution (kulapa) at Kusumapura, and, because the university of Nalanda was in Pataliputra at the time and had an astronomical observatory, it is speculated that Aryabhata might have been the head of the Nalanda university as well.[8] Aryabhata is also reputed to have set up an observatory at the Sun temple in Taregana, Bihar.[14]
WorksBiographyBorn
476 CE
Kusumapura (Pataliputra) (present-day Patna, India)[1]
Died
550 CE
Residence
India
Academic background
Influences
Surya Siddhanta
Academic work
Era
Gupta era
Main interests
Mathematics, astronomy
Notable works
Āryabhaṭīya, Arya-siddhanta
Notable ideas
Explanation of lunar eclipse and solar eclipse, rotation of Earth on its axis, reflection of light by moon, sinusoidal functions, solution of single variable quadratic equation, value of π correct to 4 decimal places, diameter of Earth, calculation of the length of sidereal year
Influenced
Lalla, Bhaskara I, Brahmagupta, Varahamihira
For his explicit mention of the relativity of motion, he also qualifies as a major early physicist.[7]
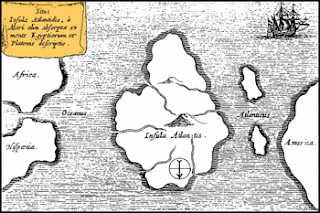
Atlantis
Despite its minor importance in Plato's work, the Atlantis story has had a considerable impact on literature. The allegorical aspect of Atlantis was taken up in utopian works of several Renaissance writers, such as Francis Bacon's New Atlantis and Thomas More's Utopia.[5][6] On the other hand, nineteenth-century amateur scholars misinterpreted Plato's narrative as historical tradition, most notably in Ignatius L. Donnelly's Atlantis: The Antediluvian World. Plato's vague indications of the time of the events—more than 9,000 years before his time[7]—and the alleged location of Atlantis—"beyond the Pillars of Hercules"—has led to much pseudoscientific speculation.[8] As a consequence, Atlantis has become a byword for any and all supposed advanced prehistoric lost civilizations and continues to inspire contemporary fiction, from comic books to films.
While present-day philologists and classicists agree on the story's fictional character,[9][10] there is still debate on what served as its inspiration. As for instance with the story of Gyges,[11] Plato is known to have freely borrowed some of his allegories and metaphors from older traditions. This led a number of scholars to investigate possible inspiration of Atlantis from Egyptian records of the Thera eruption,[12][13] the Sea Peoples invasion,[14] or the Trojan War.[15] Others have rejected this chain of tradition as implausible and insist that Plato created an entirely fictional nation as his example,[16][17][18] drawing loose inspiration from contemporary events such as the failed Athenian invasion of Sicily in 415–413 BC or the destruction of Helike in 373 BC.[19]
Tintoretto
Jacopo Comin
late September or early October 1518
Venice, Republic of Venice, Italy
Died
31 May 1594 (aged 75)
Venice, Republic of Venice, Italy
Nationality
Venetian
Known for
Painting
Movement
Renaissance, Mannerism, Venetian School
In his youth, Tintoretto was also known as Jacopo Robusti, as his father had defended the gates of Padua in a way that others called robust against the imperial troops during the War of the League of Cambrai (1509–16). His real name, "Comin", as discovered by Miguel Falomir of the Museo del Prado in Madrid, was made public on the occasion of the retrospective of Tintoretto at the Prado in 2007. The name Comin translates to the spice "cumin" in the local language.
Sunday 28 July 2019
Galileo Galilei
Born
15 February 1564
Pisa, Duchy of Florence
Died
8 January 1642 (aged 77)
Arcetri, Grand Duchy of Tuscany, Italy
Residence
Grand Duchy of Tuscany
Nationality
Italian
Alma mater
University of Pisa 1580–1585 (no degree)
Known for
Kinematics
Dynamics
Telescopic observational astronomy
Heliocentrism
Scientific career
Fields
Astronomy, physics, engineering, natural philosophy, mathematics
Institutions
University of Pisa 1589–1592
University of Padua 1592–1610
Patrons
Cardinal del Monte
Fra Paolo Sarpi
Prince Federico Cesi
Cosimo II de Medici
Ferdinando II de Medici
Maffeo Barberini
Academic advisors
Ostilio Ricci[1]
Notable students
Benedetto Castelli
Mario Guiducci
Vincenzo Viviani[2]
Signature
Galileo Galilei Signature 2.svg
Notes
His father was the musician Vincenzo Galilei. Galileo Galilei's mistress Marina Gamba (1570 – 21 August 1612?) bore him two daughters (Maria Celeste (Virginia, 1600–1634) and Livia (1601–1659), both of whom became nuns), and a son, Vincenzo (1606–1649), a lutenist.
Galileo studied speed and velocity, gravity and free fall, the principle of relativity, inertia, projectile motion and also worked in applied science and technology, describing the properties of pendulums and "hydrostatic balances", inventing the thermoscope and various military compasses, and using the telescope for scientific observations of celestial objects. His contributions to observational astronomy include the telescopic confirmation of the phases of Venus, the observation of the four largest satellites of Jupiter, the observation of Saturn's rings, and the analysis of sunspots.
15 February 1564
Pisa, Duchy of Florence
Died
8 January 1642 (aged 77)
Arcetri, Grand Duchy of Tuscany, Italy
Residence
Grand Duchy of Tuscany
Nationality
Italian
Alma mater
University of Pisa 1580–1585 (no degree)
Known for
Kinematics
Dynamics
Telescopic observational astronomy
Heliocentrism
Scientific career
Fields
Astronomy, physics, engineering, natural philosophy, mathematics
Institutions
University of Pisa 1589–1592
University of Padua 1592–1610
Patrons
Cardinal del Monte
Fra Paolo Sarpi
Prince Federico Cesi
Cosimo II de Medici
Ferdinando II de Medici
Maffeo Barberini
Academic advisors
Ostilio Ricci[1]
Notable students
Benedetto Castelli
Mario Guiducci
Vincenzo Viviani[2]
Signature
Galileo Galilei Signature 2.svg
Notes
His father was the musician Vincenzo Galilei. Galileo Galilei's mistress Marina Gamba (1570 – 21 August 1612?) bore him two daughters (Maria Celeste (Virginia, 1600–1634) and Livia (1601–1659), both of whom became nuns), and a son, Vincenzo (1606–1649), a lutenist.
Galileo studied speed and velocity, gravity and free fall, the principle of relativity, inertia, projectile motion and also worked in applied science and technology, describing the properties of pendulums and "hydrostatic balances", inventing the thermoscope and various military compasses, and using the telescope for scientific observations of celestial objects. His contributions to observational astronomy include the telescopic confirmation of the phases of Venus, the observation of the four largest satellites of Jupiter, the observation of Saturn's rings, and the analysis of sunspots.
Galileo's championing of heliocentrism and Copernicanism was controversial during his lifetime, when most subscribed to geocentric models such as the Tychonic system.[11] He met with opposition from astronomers, who doubted heliocentrism because of the absence of an observed stellar parallax.[11] The matter was investigated by the Roman Inquisition in 1615, which concluded that heliocentrism was "foolish and absurd in philosophy, and formally heretical since it explicitly contradicts in many places the sense of Holy Scripture".[11][12][13] Galileo later defended his views in Dialogue Concerning the Two Chief World Systems (1632), which appeared to attack Pope Urban VIII and thus alienated him and the Jesuits, who had both supported Galileo up until this point.[11] He was tried by the Inquisition, found "vehemently suspect of heresy", and forced to recant. He spent the rest of his life under house arrest.[14][15] While under house arrest, he wrote Two New Sciences, in which he summarized work he had done some forty years earlier on the two sciences now called kinematics and strength of materials.[16][17]Galileo Galilei (/ˌɡælɪˈleɪoʊ/ GAL-il-AY-oh, also US: /-ˈliːoʊ/ -EE-oh, Italian: [ɡaliˈlɛːo ɡaliˈlɛi]; 15 February 1564[3] – 8 January 1642) was an Italian astronomer, physicist and engineer, sometimes described as a polymath.[4] Galileo has been called the "father of observational astronomy",[5] the "father of modern physics",[6][7] the "father of the scientific method",[8] and the "father of modern science".[9][10]
In the 1920s, sculptor Gutzon Borglum and President Calvin Coolidge selected George Washington, Thomas Jefferson, Theodore Roosevelt and Abraham Lincoln to appear on Mount Rushmore—it later became an iconic symbol of presidential greatness.In political studies, surveys have been conducted in order to construct historical rankings of the success of individuals who have served as President of the United States. Ranking systems are usually based on surveys of academic historians and political scientists or popular opinion. The rankings focus on the presidential achievements, leadership qualities, failures and faults.
Subscribe to:
Posts (Atom)
Wendruff and Babcock knew almost immediately that the fossils were scorpions. But, initially, they were not sure how close these fossils ...
